It’s hard to determine whether a stock, bond, or other security is a good buy without knowing how much you can earn from holding it. But if you aren’t comfortable with math, you probably find it tedious and painful to slog through equations whenever you consider an investment.
Well, you’re in luck! The Rule of 72 is a quick way to compare stocks and bonds without stressing over the math.
What is the Rule of 72?
The Rule of 72 is an easy way to estimate how long it will take for an investment to double, given a fixed annual interest rate. By dividing 72 by the annual rate of return, you can get a rough estimate of the number of years it will take to double your initial investment.
This rule is a quick way to understand the impact of compound interest. Compound interest is the principle by which the interest you earn also earns interest. It’s an important investment concept, and it’s why you should continually reinvest your earnings. Notice how much more you can earn by holding investments that compound as opposed to those that don’t.
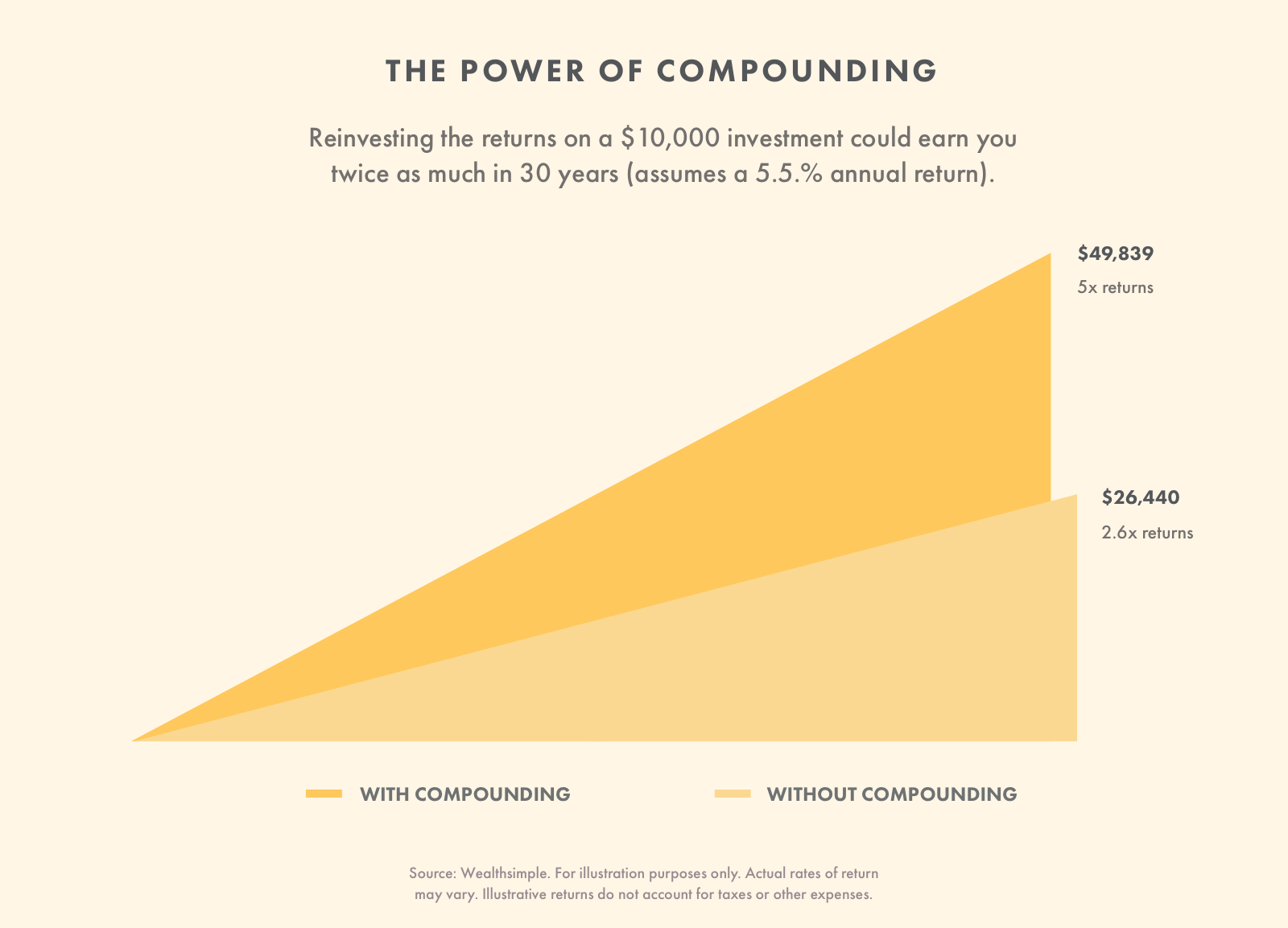
Compound interest is not easy to calculate, especially if you’re trying to do math in your head. If you earned 5% compounding interest on $1,000, you would earn $50 the first year, $52.50 the second year (which is 5% of $1,050), $55.13 the third year (which is 5% of $1,102.50), etc. The first step is easy to figure out on your own, but the rest requires a table or a complex formula.
Yes, there are countless calculators, equations, and Excel sheets that will help you determine how much you’ll earn from an investment, but the Rule of 72 is a handy mental method to gauge the investment’s approximate value. It’s perfect for some quick “napkin math” before you dive into some deeper analysis.
The Rule of 72 also creates a simple standard for comparing investments against one another. You could apply the rule to two different investments to learn which one will double in the shortest amount of time. The one that doubles first would earn the most in the long run.
Origin of the Rule of 72
The concept of interest has been around since ancient Mesopotamian, Greek, and Roman civilizations. It’s also mentioned in the Quran. It was primarily used in agriculture and land transactions, as well as standard money loans. Lenders have always needed to know how much they’ll earn from an investment.
The first reference we have of the Rule of 72 comes from Luca Pacioli, a renowned Italian mathematician. He mentions the rule in his 1494 book Summa de arithmetica, geometria, proportioni et proportionalita (“Summary of Arithmetic, Geometry, Proportions, and Proportionality”). Here’s what Pacioli says about it:
As you can see, Pacioli presents the rule in a discussion about the timeframes of doubling investments, but he doesn’t derive or explain it. We assume the rule was invented by someone else. Paciolio is just the first to mention it in a published work. Some people credit Albert Einstein for inventing the rule, but there’s no evidence to support this.
Other applications
The Rule of 72 can be used to calculate the growth of anything that’s subject to compound interest, as long as you know the rate of growth.
A country’s GDP, for example, typically increases at a compound rate. If we know the rate of growth, we can use the Rule of 72 to figure out how long it will take to double. Let’s say a country’s GDP grows at 4%. 72/4 = 18, so it would take 18 years for the GDP to double at that rate. The length of time could change, of course, if the rate of growth changes.
Inflation is another value we can look at with the Rule of 72. If money inflates at 3%, we know it will take 24 years before its value doubles. This might help you decide to invest (or not invest) in a foreign currency or foreign bond.
In fact, we can use the Rule of 72 to look at more than just financial concepts. We can estimate when anything will double if we know the growth rate. For instance, you could predict when a population would double. If you know the population grows by 5%, it would double in 14.4 years.
Rule of 72 formula
Calculating the rule of 72 is remarkably simple. Unlike most investment equations, you don’t need to know a complex formula. In some cases, you can perform this calculation in your head.
The estimated number of years to double an investment = 72 / compound annual interest rate
Note: You must use the annual interest rate as a whole number, not a decimal (e.g., “5”).
The Rule of 72 isn’t perfectly accurate, but it’s reasonably accurate for low rates of return. Since we’re doing quick-and-dirty math here, it’s accurate enough for our purposes. The following chart compares the results from the Rule of 72 versus the actual number of years it will take to double the investment.
Rate of Return | Years according to the Rule of 72 | Actual number of years | Difference of years |
|---|---|---|---|
| 1% | 72 | 72 | 0 |
| 2% | 36 | 35 | 1 |
| 3% | 24 | 23.45 | 0.6 |
| 5% | 14.4 | 14.21 | 0.2 |
| 7% | 10.3 | 10.24 | 0 |
| 9% | 8 | 8.04 | 0 |
| 12% | 6 | 6.12 | 0.1 |
| 25% | 2.9 | 3.11 | 0.2 |
| 50% | 1.4 | 1.71 | 0.3 |
| 72% | 1 | 1.28 | 0.3 |
| 100% | 0.7 | 1 | 0.3 |
As you can see, the Rule of 72 gets us pretty close to the exact number of years it would take to double an investment. The rule becomes less accurate as the interest rate gets higher, but it’s exceptionally accurate up to about 10%. Few investments receive higher returns than that, so the rule works in most practical cases.
You can also invert the Rule of 72 to help you determine the interest rate you’ll need if you want to double your investment within a certain amount of time. This is as easy as flipping the equation around.
Interest rate = 72 / Years to double an investment
Let’s say you want to double your investment in eight years. 72 / 8 = 9, so you would need to earn 9% in order to double your investment within that window. This is a clever technique you can use to determine where to invest your money in order to meet your financial goals.
Rule of 72 example
Acme Technology Fund is an ETF that invests in U.S. tech companies that show moderate growth. The ETF has earned a consistent 8% per year for ten years. They have no intention of changing the fund’s criteria, so you are reasonably sure they will continue to see 8% growth going forward. (You don’t know for sure, of course, but you’re as confident as you can be.)
If you purchase shares of the Acme Technology Fund, how long will it take to double your money?
72 / 8 = 9 years to double the original investment
To be fair, it would technically take 9.01 years to double an investment at 8% interest. Like we said earlier, the Rule of 72 isn’t perfectly accurate, but it’s close enough to be useful. You would come up with the exact figure if you used the complete compound interest formula.
Now let’s say you’re looking for an investment that will double your money. You’d like to double it within 12 years. What’s the minimum interest rate you would need to hit that goal?
72 / 12 = 6%
In this case, you would need to find an investment that can reliably achieve 6% growth every year. Higher is better, of course, but if the investment is lower than 6%, you won’t achieve your goal of doubling your money in 12 years.
Does the Rule of 72 work?
The Rule of 72 helps you estimate how quickly an investment will double. It’s a mathematical test that simply compares two numbers together. So in that sense, it always works. Keep in mind this number is an estimation. It grows less accurate as the interest rate increases due to the inaccuracies we explained above.
But the Rule of 72 cannot predict the future. The rule can’t guarantee that an investment will continue to rise at the interest rate you input. In fact, nobody can guarantee how an investment will perform in the future. There are countless variables that can affect an investment’s performance, none of which are considered by the rule.
This means the Rule of 72 shouldn’t be the end of your analysis. You should conduct a thorough review of any security you buy to get some understanding as to whether it will continue to maintain its growth rate.
Or better yet, don’t worry about evaluating stocks and bonds to find ones that grow your money. Use automated investing to boost your worth using an intelligent portfolio of low-fee funds that are designed to meet your financial goals. If you don’t have the stomach to risk your money (because all investing comes with some risk), drop your nest egg in a high interest savings account.


